James L. Hilton
U.S. Naval Observatory, 3450 Massachusetts Ave., NW, Washington, DC 20392
A new set of ephemerides for 15 of the largest asteroids, USNO/AE98, has been produced for use in the Astronomical Almanac. The ephemerides cover the period from 1800 through 2150. The internal uncertainty in the mean longitude at epoch ranges from 0."05 for 7 Iris through 0."22 for 65 Cybele and the uncertainty in the mean motion varies from 0."02 cen.-1 for 4 Vesta to 0."14 cen.-1 for 511 Davida. This compares favorably with the internal errors for the outer planets in DE200. However, because the asteroids have relatively little mass and are subject to perturbations by other asteroids the actual uncertainties in their mean motions are likely to be a few tenths of an arcsecond per century.
As part of improving the ephemerides, new masses and densities were determined for 1 Ceres, 2 Pallas, and 4 Vesta, the three largest asteroids. These masses are: Ceres = (4.39 ± 0.04) × 10-10 MSun, Pallas = (1.59 ± 0.05) × 10-10 MSun, Vesta = (1.69 ± 0.11) × 10-10 MSun. The mass for Ceres is smaller than most previous determinations of its mass. This smaller mass is a direct consequence of the increase in the mass determined for Pallas. The densities found are 2.05 ± 0.05 gm. cm-3 for Ceres, 4.2 ± 0.3 gm cm-3 for Pallas and 4.3 ± 0.3 gm. cm-3 for Vesta. The density for Ceres is somewhat greater than that found for the taxonomically similar 253 Mathilde.
Comparison of the USNO/AE98 ephemerides of Ceres, Pallas, Juno, and Vesta with the Duncombe (1969) shows some differences that are directly attributable to the inclusion of asteroid perturbations in the physical model.
This document is designed for the reader who wants to know the details of the reduction of the data and determination of the ephemerides. A paper summarizing the process for the general reader is in preparation.
The Astronomical Almanac has published ephemerides for 1 Ceres, 2 Pallas, 3 Juno, and 4 Vesta since its edition for 1953. Historically, these four asteroids have been observed more than any of the others. Even in modern times few asteroids have had more attention paid to them. Ceres, Pallas, and Vesta deserve such attention because they are the three most massive asteroids, the source of significant perturbations of the planets, and are among the brightest main belt asteroids making them prime targets for photometric and spectroscopic studies aimed at understanding the composition of the asteroids. They are also the largest asteroids in diameter, so they have been the subjects of diameter determinations by groups such as Millis, et al. (1987), Lambert (1985), Magnuson (1986), Drummond & Cocke (1988), and Thomas, et al. (1997).
The ephemerides currently published in the Astronomical Almanac are based on the dated work of (Duncombe 1969). These ephemerides extend only until January 7, 2000. As a result, the U.S. Naval Observatory has produced a new set of ephemerides for use in the Astronomical Almanac.
Interest in the asteroids has increased significantly over the past several years for many reasons such as looking for clues to the origin and primordial composition of the solar system, the dynamics of small solar system bodies, and the potential of asteroid collisions with the Earth. The asteroids are also a source of significant perturbations of the major planets. DE200, the JPL planetary ephemerides currently used in the Astronomical Almanac was constructed using perturbations from five asteroids (Standish 1990) while more recent planetary ephemerides such as DE403 include perturbations from 300 asteroids (Standish, et al. 1995). Thus, it was decided to include more than the traditional four asteroids in the production of asteroid ephemerides. The criteria used to select a small sample of main belt asteroids for producing ephemerides were:
| Asteroid | Diameter > 300 km | Observed before 1850 | Largest in Class |
|---|---|---|---|
| 1 Ceres | 933a | X | X |
| 2 Pallas | 525b | X | |
| 3 Juno | X | ||
| 4 Vesta | 530c | X | X |
| 6 Hebe | X | ||
| 7 Iris | X | ||
| 8 Flora | X | ||
| 9 Metis | X | ||
| 10 Hygiea | 407d | X | |
| 15 Eunomia | X | ||
| 16 Psyche | X | ||
| 52 Europa | 302d | ||
| 65 Cybele | 310e | ||
| 511 Davida | 326d | ||
| 704 Interamnia | 317d |
These 15 asteroids make up the USNO/AE98 (U.S. Naval Observatory Asteroid Ephemerides of 1998). The USNO/AE98 covers the period 1799 November 16 (JD 2378450.5) through 2100 February 1 (JD 2488100.5).
The construction of the ephemerides is discussed in the following sections.
Data discusses the sources of the data used to determine
the ephemerides and how the data was handled.
Physical Model discusses the physical model used
to integrate the ephemerides. Asteroid Masses
discusses the masses and densities of the largest asteroids.
Ephemerides discusses the resulting ephemerides.
And Residuals looks at the residuals and places
limits on the accuracy of the ephemerides.
Data
An ephemeris is only as good as the data and the physical model that are used to generate it. The ephemerides of the asteroids are based on optical positions, like the ephemerides of the outer solar system planets. However, there are also two radar delay observations of main belt asteroids. Bowell et al. (1996) finds a simple relation between the uncertainty in the osculating elements depending primarily upon the length of time over which the the asteroid has been observed and the number of oppositions at which observations have been made. However, for over sampled asteroids such as Ceres, Pallas, Juno, and Vesta the uncertainty in the ephemerides can be further reduced based on the number and quality of observations. Thus an idea of the currently best available ephemeris accuracy and accuracy reachable can be inferred from Table 2.
| Asteroid | Year Discovered | Oppositions as of April 1997 | Years Covered | Oppositions Covered | Observations | Reference |
|---|---|---|---|---|---|---|
| 1 Ceres | 1801 | 152 | 1839-1992 | 62 | 4676 | Bowell (1994a) |
| 2 Pallas | 1802 | 151 | 1839-1993 | 63 | 5482 | Bowell (1994b) |
| 3 Juno | 1804 | 148 | 1839-1993 | 65 | 4741 | Bowell (1994b) |
| 4 Vesta | 1807 | 136 | 1841-1992 | 56 | 5168 | Bowell (1994a) |
| 6 Hebe | 1847 | 110 | 1869-1994 | 59 | 702 | Bowell (1994a) |
| 7 Iris | 1847 | 109 | 1850-1993 | 59 | 3313 | Bowell (1994b) |
| 8 Flora | 1847 | 103 | 1850-1993 | 57 | 859 | Bowell (1994b) |
| 9 Metis | 1848 | 107 | 1849-1993 | 50 | 815 | Bowell (1994c) |
| 10 Hygiea | 1849 | 120 | 1849-1993 | 59 | 1247 | Bowell (1994a) |
| 15 Eunomia | 1851 | 111 | 1869-1993 | 50 | 775 | Bowell (1994a) |
| 16 Psyche | 1852 | 116 | 1870-1994 | 55 | 976 | Bowell (1994a) |
| 52 Europa | 1858 | 112 | 1902-1994 | 53 | 761 | Bowell (1994c) |
| 65 Cybele | 1861 | 113 | 1870-1993 | 50 | 447 | Bowell (1994c) |
| 511 Davida | 1903 | 76 | 1903-1993 | 70 | 930 | Goffin (1993a) |
| 704 Interamnia | 1910 | 69 | 1910-1992 | 58 | 1625 | Goffin (1993b) |
The optical observations used in creating these ephemerides came from two data types: wide angle data (mainly from transit instruments) and relative data (positions measured relative to nearby background stars). Each of these data types are handled differently, so their sources and methods of reduction will be discussed separately.
Some aspects of the data reduction was handled using the ephemeris program PEP
(Ash 1965). PEP is a high accuracy ephemeris generating
program capable of of generating ephemerides using complicated physical models,
comparing the results to many different observation types, adjusting designated
parameters and then producing a new set of ephemerides. PEP can iterate the
ephemerides until a desired level convergence in the model parameters is
reached. In addition to adjusting physical model parameters, PEP can adjust
such parameters as catalog corrections in right ascension and declination,
electronic delay biases in delay-Doppler observations, and corrections in the
location of observatories. PEP can also be used to determine the
root-mean-square uncertainty in a set of observations for use in weighting
those observations.
WIDE ANGLE DATA
This data is usually taken with a transit instrument. The measurements are not
made with respect to nearby stars, but are measured by means such as time of
transit or recorded using setting circles. Usually the data is referred to an
equator and equinox determined from the measurements made by the instrument
itself, that is a fundamental catalog, but occasionally, as in the case of the
Carlsberg Meridian Circle,
(Morrison et al, 1990) the observations are
differentially reduced to a preexisting equator and equinox. The positions
for these observations are usually given as a geocentric apparent position with
respect to the equator and equinox of date.
Most asteroid observations from the nineteenth century are of the wide angle, fundamental catalog variety. The main sources of these observations are the Royal Greenwich Observatory (Maskelyne 1811; Pond 1815-1835; Airy 1837-1883; Christie 1884-1902; Schubart 1976), l'Observatoire de Paris (Le Verrier 1858-1867; l'Observatoire de Paris 1871; Mouchez 1880-1892; Loewy 1898-1907; Baillaud 1910-1911; Schubart 1976), the Royal Observatory, Edinburgh (Henderson 1839-1847; Henderson & Smythe 1848-1852; Schubart 1976), the Cambridge Observatory (Airy 1831-1836; Challis 1837-1864; Adams 1879; Schubart 1976), the Royal Observatory, Cape of Good Hope (MacLear & Stone 1871-1872; MacLear & Gill 1900), the Sternwarte zu Kremsmunster (Koller 1833-1839; Reslhuber 1841-1870; Strasser 1870-1880), and the U.S. Naval Observatory (Gillis 1846; Maury 1846-1859; Gillis 1867; Yarnall et al. 1872; U.S. Naval Observatory 1906b; Harkness & Skinner 1900). An additional 4299 observations from other observatories were gathered from the Astronomische Nachrichten (1832-1900) and early observations of Ceres and Pallas made at Palermo, Milan and Seeburg were provided by Schubart 1976. In all, nineteenth century wide angle observations were gathered from 39 observatories. Except for the later USNO observations (Yarnall et al. 1872; U.S. Naval Observatory 1906b; Harkness & Skinner 1900) the data was not collected into comprehensive catalogs, but was reduced at yearly intervals.
Aside from Ceres, Pallas, Juno, and Vesta, very few wide angle observations were made of asteroids from 1901 until 1985. There are only a few observatories to make wide angle observations. The wide angle asteroid observations for the twentieth century used were provided by the Royal Greenwich Observatory (Blackwell et al. 1975; Buontempo et al. 1973; Christie 1903-1912; Dyson 1913-1933; Dyson & Jones 1934; Jones 1935-1953; Tucker et al. 1983), the Cape Observatory (Stoy 1968), the U.S. Naval Observatory (Adams et al. 1964; Adams & Scott 1968; Hughes & Scott 1982; Hughes et al. 1992; U.S. Naval Observatory 1906a; Watts & Adams 1949), the Carlsberg meridian circle (Carlsberg Meridian Catalog 1984-1995), and the Universite de Bordeaux transit circle (Minor Planet Center 1997). Like the nineteenth century data, the Greenwich data prior to 1940 was reduced on a yearly basis. However, the data from the U.S. Naval Observatory, the Cape Observatory, and the Royal Greenwich Observatory after 1940 were reduced to fundamental catalogs that were the result of multi-year observing programs. The Carlsberg meridian circle data was also reduced to apparent positions, but on the system of the FK5 rather than as a fundamental catalog ( Morrison et al. 1990). The Universite de Bordeaux data, taken from the Minor Planet Center, has been reduced to topocentric astrometric positions on the mean equator and equinox of J2000.0.
Another potential source of wide-angle data was the Hipparcos astrometric satellite. Hipparcos asteroid observations were examined for inclusion in the ephemerides. However, the Hipparcos observations are one dimensional observations made along a great circle at an arbitrary inclination with respect to the celestial equator. The span of the Hipparcos mission was only 3.3 years, so the usefulness of the observations is limited despite the high one-dimensional accuracy of the observations. For this reason the Hipparcos observations were not used.
Data through the late nineteenth century was made using micrometers to compare the asteroid with one or two field stars. Essentially, the position was calculated at the telescope.
Beginning in the late nineteenth century photographic plates were used to record the images of the asteroid and the background stars. These plates were later measured and a position calculated for the asteroid. Photographic plates have the added advantage of being able to collect light for extended periods of time allowing objects too dim to be viewed directly to be recorded. For this reason photographic plates have been the dominant form of recording asteroid positions through most of the twentieth century. Initially, only three or so stars were measured to determine the asteroid's position because the arithmetic needed to produce a position could become quite arduous as more background objects are used to determine the asteroid position. As computers became more commonplace, the arithmetic became less of a burden so more stars could be measured and more sophisticated reduction models could be implemented.
Modern relative positions are most often made using CCDs. A CCD produces a permanent record like photographic plates, and has the added advantage of consisting of an array of digital pixels allowing a more highly accurate determination of the center of light of the asteroid and the background objects. The main drawback to using a CCD is it has a much smaller field of view than a photographic plate. Thus reduction of CCD observations require the use of star catalogs with a much higher magnitude limit to provide enough stars to determine the asteroid position.
The observations were originally reduced to the dynamical coordinate system at a variety of epochs using several different methods. They also use positions of stars published in a variety of catalogs with varying degrees of accuracy. As a result, only the most recent relative position observations are of an accuracy comparable to that of the wide-angle observations.
The source for most of the relative observations used was the Minor Planet Center (1997). There are two advantages to using the Minor Planet Center data rather than collecting it from its original sources. First, the data is collected in a single place saving time. Second, unlike wide angle data which is reduced to apparent position of date, relative data is reduced to an epoch. The Minor Planet Center has provided the transformation from the original epoch of publication to the epoch of J2000.0. A total of 35,575 relative observations from 131 observatories were included.
Herget (1947) required that all observations submitted to the MPC be on the B1950.0 coordinate system. Earlier observations have been collected by the MPC and converted to B1950.0 coordinates using the information available along with the published observations. In January 1992 when the MPC switched over to the J2000.0 coordinate system (Marsden 1991a). The MPC then converted the positions of all of the observations it had at that time from the B1950.0 to the FK5/J2000.0 coordinate system using the procedures given on pages B42 and B43 of the Astronomical Almanac, taking into account that these are asteroids and not stars (Marsden 1991b).
However, it has always been the responsibility of the observer to reduce the observations to the required coordinate system. Thus it was necessary to subject all observations, both relative and wide-angle to scrutiny to make sure that there were no significant errors in the data. How this was done is described in Data Weighting.
One other source of relative astronomical data was Stone (1995 - 1997a) at the U.S. Naval Observatory, Flagstaff Station. The technique used for these high precision observations is described in Stone (1997b). These data were extremely valuable because they provided very high accuracy data at the most recent opposition of the asteroids. This provides a very solid anchor point for the modern end of the asteroid observations.
Radar data is also complementary to optical data. Rather than producing a place on the sky it produces a time delay or distance to the object and the component of the velocity along the line of sight. As a result, radar data has been shown able to produce great improvements in asteroid ephemerides for those asteroids with a rather small number of observations ( Yeomans et al. 1992, Hilton 1997).
Until the recent upgrade of the Arecibo radio telescope, radar observations of all but the largest main belt asteroids has been impossible. To date the only time delay-Doppler observations published for the asteroids whose ephemerides are determined here are two observations of Iris by Ostro et al. (1991). These data were included in the ephemeris of Iris. Because the amount and span of other data on Iris is large and the uncertainty in the time delay was rather large (42 km and 24 km) the contribution of the radar data to the ephemerides was rather small. The change in the orbital parameters was about 0.02 sigma and the reduction in the formal uncertainties in the orbital parameters was about 0.01 sigma in the final solution.
First, the observations from each observatory were divided up into logical groups. Several criteria were used to determine what constituted a logical group. Easiest to determine were those observations that were published as part of a catalog. These data formed the bulk of the twentieth century, wide angle observations. The next criterion was for known changes of equipment, reduction technique, catalog used for reduction, or position of an observatory. Next the records of each of the observatories were examined. If the observations from an observatory clustered around a set of epochs, those observation near each epoch were identified as a logical group. Finally, in absence of no other information, groups were devised by dividing data into groups at points where changes of equipment or techniques, such as the introduction of the impersonal micrometer and atomic time, were known to have been widely adopted.
The observations were then examined for relevance to the final solution. Unless the observations of an asteroid from an observatory met one of the following conditions, they were dropped from the final solution:
| Asteroid | Year of Last Opposition Covered | Observations in Right Ascension | Observations in Declination | Total Observations | Oppositions Covered |
|---|---|---|---|---|---|
| 1 Ceres | 1996 | 9229 | 9031 | 9354 | 139 |
| 2 Pallas | 1996 | 9068 | 8907 | 9205 | 138 |
| 3 Juno | 1996 | 7617 | 7481 | 7751 | 124 |
| 4 Vesta | 1996 | 10,324 | 10,087 | 10,475 | 131 |
| 6 Hebe | 1997 | 4701 | 4521 | 4737 | 93 |
| 7 Iris | 1997 | 4478 | 4279 | 4547 | 85 |
| 8 Flora | 1995 | 2190 | 1898 | 2247 | 82 |
| 9 Metis | 1995 | 1982 | 1724 | 2033 | 68 |
| 10 Hygiea | 1996 | 2009 | 1949 | 2035 | 87 |
| 15 Eunomia | 1996 | 1583 | 1357 | 1610 | 70 |
| 16 Psyche | 1997 | 1590 | 1526 | 1620 | 80 |
| 52 Europa | 1996 | 1145 | 1123 | 1156 | 72 |
| 65 Cybele | 1996 | 729 | 731 | 736 | 78 |
| 511 Davida | 1996 | 671 | 673 | 677 | 64 |
| 704 Interamnia | 1996 | 1392 | 1396 | 1398 | 53 |
All of the asteroids used data from their first observed opposition through the most recent opposition given in Table 3. Except for Davida and Interamnia all of the asteroids are fit to substantially more data over substantially more oppositions than the ephemerides described in Table 2. As described in the section on Juno, it was fit to 7318 observations in right ascension and 7174 observations in declination of a total 7428 observations covering 111 oppositions from 1839 through 1996 because there are unaccounted for perturbations on Juno. Observations from 1804 through 1838 were rejected.
The next step was to determine the root-mean-square error for each logical
grouping. A single "catalog" root-mean-square error was produced for all of
the asteroids observed at an observatory over the time span of a logical
grouping. An a priori root-mean-square error was assigned based on the age of
the observation and the technique used for obtaining it. Initial ephemerides
were constructed and the difference between the a priori error and the actual
root-mean-square error for each logical group was examined to determine the
adjustment in the error. Iterating with a new ephemerides calculated using the
new root-mean-square errors was possible, but in all cases a single iteration
was sufficient to determine the root-mean-square error of the observations.
For those catalogs contributing 500 or more observations to the ephemerides
corrections to the equinox and declination were also calculated. Corrections
to the equinox and declination were applied to 70% of the relative observations
and 88% of the wide-angle observations. Smaller data sets were examined for
deviation from zero in the mean residuals. In all cases the corrections were
less than 1" and less than 1/5 of the root-mean-square error for any group of
observations, so they did not significantly affect either the root-mean-square
error or the final ephemerides. Smaller data sets were examined for deviation
from zero in the mean residuals. The root-mean-square error was then used to
directly weight the observations in each catalog. Generally, except for the
modern CCD observations, the relative observations were a factor of 2 worse
than wide angle observations for a similar epoch.
Physical Model
The Planetary Ephemeris Program, PEP, is the software used for generating the asteroid ephemerides (Ash 1965). PEP is a high accuracy program capable of generating ephemerides using complicated physical models, comparing the results to many different observation types, adjusting designated parameters and then producing a new set of ephemerides. PEP can iterate the ephemerides until a desired level of convergence in the model parameters is reached. Standish (1987) compared a set of PEP generated ephemerides with similar JPL ephemerides and found the differences between those ephemerides were less than the uncertainties in the ephemerides. In addition to adjusting physical model parameters, PEP can adjust such parameters as catalog corrections in right ascension and declination, electronic delay biases in delay-Doppler observations, and corrections in the location of observatories. The final ephemerides were integrated using PEP's Adams-Moulton integrator with a step size of 2 days. The epoch of integration was 18 December 1997 (JD 2450800.5).
Asteroid perturbations are the largest source of incompletely modeled perturbations of the planets especially Mars and the Earth-Moon barycenter. Williams (1984) shows no less than seven asteroids capable of making periodic perturbations of more than a kilometer to Mars. The largest asteroid, Ceres, has only 0.13% the mass of Mars and is located within the asteroid belt itself. Hence it and the other asteroids are much more sensitive to the perturbations of the asteroids than are the planets. Thus the physical model for the asteroid ephemerides need to include perturbation by other asteroids.
| Asteroid | Perturbing Asteroids |
|---|---|
| 1 Ceres | Pallas, Vesta |
| 2 Pallas | Ceres, Vesta |
| 3 Juno | Ceres, Pallas, Vesta, Psyche, Davida |
| 4 Vesta | Ceres, Pallas |
| 6 Hebe | Ceres, Pallas, Vesta |
| 7 Iris | Ceres, Pallas, Vesta |
| 8 Flora | Ceres, Pallas, Vesta |
| 9 Metis | Ceres, Pallas, Vesta |
| 10 Hygiea | Ceres, Pallas, Vesta |
| 15 Eunomia | Ceres, Pallas, Vesta, Davida |
| 16 Psyche | Ceres, Pallas, Juno, Vesta |
| 52 Europa | Ceres, Pallas, Vesta |
| 65 Cybele | Ceres, Pallas, Vesta |
| 511 Davida | Ceres, Pallas, Vesta, Eunomia, Interamnia |
| 704 Interamnia | Ceres, Pallas, Vesta, Davida |
The perturbing asteroids included in the ephemeris of each asteroid are given in Table 4.The mass for Interamnia was the mean of two values determined by Landgraff (1992). The mass for Davida is the estimate used by Viateau & Rapaport(1997). The mass for Eunomia was taken from Hilton (1997). The masses for Juno and Psyche were estimated from their Tedesco (1989) diameters and an assumed density of 3 g cm-1. The masses of Ceres, Pallas, and Vesta were determined contemporaneously with the ephemerides. The final masses determined in a simultaneous solution are in Table 5 and discussed in Asteroid Masses.
| Asteroid | Perturbed Asteroid | Mass (10-10 MSun) |
|---|---|---|
| 1 Ceres | Pallas, Vesta | 4.39 ± 0.04 |
| 2 Pallas | Ceres | 1.59 ± 0.05 |
| 4 Vesta | Ceres | 1.69 ± 0.11 |
| Perturbing Asteroid | Perturbed Asteroid | Mass (10-10 MSun) |
|---|---|---|
| 1 Ceres | Junoa,b | 4.69 ± 0.27 |
| 1 Ceres | Vestaa,b | 4.82 ± 0.18 |
| 1 Ceres | Pallasb | 4.37 ± 0.07 |
| 1 Ceres | Junob,c | 4.15 ± 0.27 |
| 1 Ceres | Vestab,c | 4.50 ± 0.18 |
| 1 Ceres | Pallas, Vestad | 4.35 ± 0.05 |
| 1 Ceres | Pallas, Vestae | 4.39 ± 0.04 |
| 2 Pallas | Ceresb | 1.57 ± 0.06 |
| 2 Pallas | Ceresd | 1.60 ± 0.04 |
| 2 Pallas | Cerese | 1.59 ± 0.05 |
| 4 Vesta | Ceresb | 1.52 ± 0.15 |
| 4 Vesta | 197 Arete | 1.58 ± 0.11 |
| 4 Vesta | Ceresd | 1.52 ± 0.09 |
| 4 Vesta | Cerese | 1.69 ± 0.11 |
 |
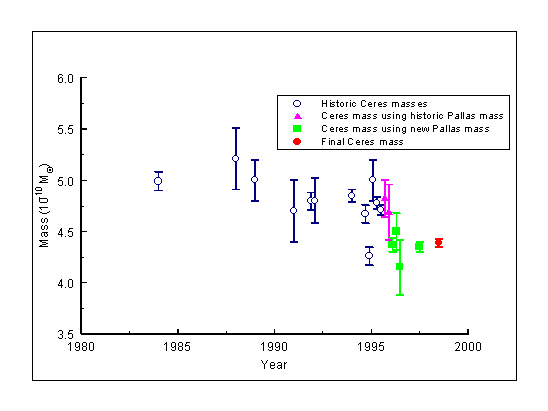
| Figure 1. The history of mass determinations of 1 Ceres. |
Why are these masses for Ceres so dependent on the mass of Pallas? The answer is that there is a degeneracy between the masses of Pallas and Ceres when fitting observations to an orbit over a long period of time. The reason for this degeneracy is that the mean distances of Ceres and Pallas are very similar (the synodic period of Ceres and Pallas, based on Williams (1989) proper semi-major axes, is 2000 yr.) and Ceres and Pallas have similar mean longitudes (increasing from approximately 1° at the time of discovery of Pallas to 43° in Dec. 1997). As a result, if the mass of Pallas is fixed at a wrong value difference in the perturbations of Pallas are propagated into the mass of Ceres. The earliest determinations of the mass of Ceres were made using Pallas as the perturbed body, but suffered from systematic errors. Figure 2 shows the history of the values determined for the mass of Pallas. The mass determined here is about 1.5 sigma greater than the two most recent values for the mass using Ceres as the perturbed body; however, it is in good agreement with the mass determined using the Viking lander data, in red. The blue point near the final mass in green was a preliminary mass determined using approximately half the data in the final solution. As Figure 1 shows, using the historic values for the mass of Pallas brings the mass of Ceres into perfect agreement with most of the recent determinations of the mass of Ceres. Thus it is the difference in the mass determined for Pallas that changes the mass determined for Ceres. In the case of Kuzmanoski (1995) the act of treating the encounter as an impulse allowed the author to look only at the immediate effect of Ceres on Pompeja and ignored the long term effect of Pallas inherent in the traditional fitting the data to an improved physical model approach.
Ceres' orbit is mildly eccentric (0.097) and inclined 9°.7 to the ecliptic, while Pallas' orbit is more eccentric (0.180) and inclined 35°.7 to the ecliptic. Hence Ceres and Pallas are physically close only at the nodes of their orbits, even though they have similar mean distances and mean longitudes. This does not affect the degeneracy in determining the masses of Ceres and Pallas, however, because the observed effect is the average perturbation of Pallas over several orbits.
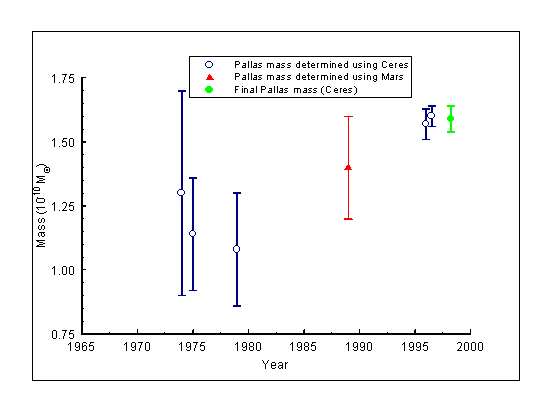 |
| Figure 2. The history of mass determinations of 2 Pallas. |
The one existing alternative technique is Standish & Hellings (1989). Here the masses for Ceres, Pallas, and Vesta are determined using the Viking lander ranging data. Just as the mass of Pallas found here is nearly 1 sigma above the Standish & Hellings mass, the mass for Ceres is nearly 1 sigma smaller, reflecting the degeneracy in determining their masses. However, Standish, et al. (1995) reverted to a lower mass for Pallas for DE403 without explanation.
Looking for other perturbed asteroids is difficult because Pallas is in a
highly inclined, eccentric orbit, reducing the number of close encounters.
Those close encounters that do occur are usually at high velocity, reducing the
size of the perturbation caused by Pallas. The best candidate found, so far,
is 2495 Noviomagnum which encountered Pallas on 1 Jan. 1991 at a minimum
distance of 0.036 AU (Hilton, et al. 1996).
Unfortunately, fitting to existing observations with Pallas as a perturbing
body changes the right ascension by only 0."12 twenty years after the encounter
when compared to ephemerides generated without Pallas as a perturbing body.
VESTA
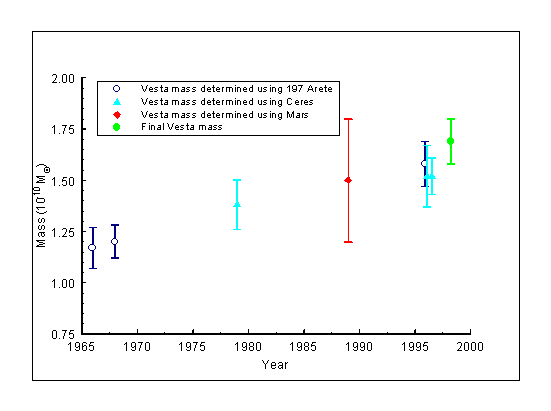 |
| Figure 3. The history of mass determinations of 4 Vesta. |
The final masses here were determined using a simultaneous solution for the
masses of all three asteroids. Masses for all three asteroids were determined
using a variety of observation data sets and initial conditions. The masses of
Ceres and Vesta were also determined using other perturbed asteroids. Aside
from the dependence of the mass determined for Ceres on the mass used for
Pallas, all of the masses are robust. The masses determined in all other cases
were within 1.5 sigma of the final masses. Thus, the mass uncertainties
are quite realistic.
ASTEROID DENSITIES
New masses for Ceres, Pallas, and Vesta also give new opportunities for
determining the densities of asteroids.
The volume of Ceres is based on the observation of a stellar occultation of BD+8° 471 by Ceres (Millis et al. 1987). The equatorial radius was determined to be 479.6 ± 2.4 km and the polar radius is 466.3 ± 4.5 km. Assuming that Ceres is nearly spheroidal in shape the mean radius is then 466.3 ± 5.7 km. This mean radius is in very good agreement with Hubble Space Telescope (HST) images of Ceres (Merline et al. 1996). The area of Ceres was found to consist of 240 pixels with a linear dimension of 53 km. The mean radius from the HST images is thus 463 km. The derived volume for Ceres is (42.5 ± 0.9) × 107 km3 which gives a mean density of Ceres is 2.05 ± 0.05 gm cm-3. This density is significantly greater than the density of the taxonomically similar 253 Mathilde (volume 80000 ± 12000 km3 and density 1.3 ± 0.2 gm cm-3}) (Veverka et al. 1997). Since Ceres is nearly 8500 times more massive than Mathilde, the difference in the density could be caused by greater compaction or a minimal amount of differentiation rather than a major difference in composition. Or, since Ceres is a G-type asteroid (considered a sub-type of the C-type asteroids) while Mathilde is a C-type asteroid (Tholen 1989), compositional differences may account for the difference in density.
No HST images for Pallas exist. However, there are several determinations of Pallas' shape based on stellar occultations and speckle interferometry ( Lambert, 1985; Magnuson, 1986; Drummond & Cocke, 1988). All of these papers yield similar results. For the final density calculation, the spheroid of Drummond & Cocke, 570 ± 22 × 525 ± 4 × 482 ± 15 km, was used. This spheroid is based on a combination of three stellar occultations and speckle interferometry. The derived volume of Pallas is (7.6 ± 0.4) × 107 km3. The density is 4.2 ± 0.3 gm cm-3.
Thomas, et al. (1997) have determined a mean radius for Vesta of 265 ± 5 km which gives a volume of (7.8 ± 0.3) × 107 km3. The derived density is 4.3 ± 0.3 gm. cm-3.
The integration of the asteroid orbits, computation of the (O - C)s, and adjustment of parameters to produce the ephemerides was carried out using PEP. The adjusted parameters in the solution were the osculating elements of the asteroids, the masses of Ceres, Pallas, and Vesta, and the catalog corrections for the 49 catalogs of observations that contributed 500 or more observations to the asteroid ephemerides. The total number of adjusted parameters was 191. The epoch of integration was 18 Dec. 1997 (JD 2450800.5).
The determination of catalog corrections had little effect upon the ephemerides generated. In all cases the adjustment was less than 1" and a factor of 1/5 or less of the mean uncertainty in the observations. Not adjusting the catalog corrections changed the ephemerides by only 10-6 AU in semi-major axis, 10-7 in eccentricity, and 0."004 in the angular elements in the most extreme case. The change in apparent position on June 21.5, 1800 between the ephemeris with catalog corrections and that without catalog corrections is 0."5 or about 17% of the root-mean-square uncertainty of observations from that time period.
The final ephemerides generated for all fifteen asteroids were generated covering the period November 16, 1799 (JD 2378450.5) through February 1, 2100 (JD 2488100.5) with a tabular interval of 2 days. The ephemerides give the position and velocity of each asteroid in equatorial rectangular coordinates on the mean equator and equinox of J2000.0. The positions are given in Astronomical Units (AU) and the velocities are in AU day-1.
The osculating equatorial elements for the asteroids and their formal uncertainties for the final ephemerides are given in Table 7. The epoch for the elements is Dec. 18, 1997 (JD 2540800.5).
Asteroid Element Value Uncertainty Units
1 Ceres mean distance 2.767837933 2 × 10-9 AU
eccentricity 0.07741186 2 × 10-8
inclination 27.143874 2 × 10-6 degrees
ascending node 23.390576 4 × 10-6 degrees
argument of perihelion 132.77714 1 × 10-5 degrees
mean anomaly 207.08207 1 × 10-5 degrees
2 Pallas mean distance 2.773856966 2 × 10-9 AU
eccentricity 0.23233958 2 × 10-8
inclination 11.833838 2 × 10-6 degrees
ascending node 160.858815 9 × 10-6 degrees
argument of perihelion 323.02675 1 × 10-5 degrees
mean anomaly 194.831730 6 × 10-6 degrees
3 Juno mean distance 2.669481231 1 × 10-9 AU
eccentricity 0.25773186 2 × 10-8
inclination 10.876713 2 × 10-6 degrees
ascending node 11.70013 1 × 10-5 degrees
argument of perihelion 46.91848 1 × 10-5 degrees
mean anomaly 72.053979 4 × 10-5 degrees
4 Vesta mean distance 2.3607012365 7 × 10-10 AU
eccentricity 0.09035411 1 × 10-8
inclination 22.735663 2 × 10-6 degrees
ascending node 18.172807 4 × 10-6 degrees
argument of perihelion 237.07613 1 × 10-5 degrees
mean anomaly 138.49976 1 × 10-5 degrees
6 Hebe mean distance 2.424774096 1 × 10-9 AU
eccentricity 0.20184319 3 × 10-8
inclination 15.512280 2 × 10-6 degrees
ascending node 38.815450 9 × 10-6 degrees
argument of perihelion 341.13194 1 × 10-5 degrees
mean anomaly 211.606826 7 × 10-6 degrees
7 Iris mean distance 2.384906313 1 × 10-9 AU
eccentricity 0.23063285 2 × 10-8
inclination 23.084891 2 × 10-6 degrees
ascending node 346.012381 6 × 10-6 degrees
argument of perihelion 57.998014 8 × 10-6 degrees
mean anomaly 214.061635 6 × 10-6 degrees
8 Flora mean distance 2.201299304 1 × 10-9 AU
eccentricity 0.15606734 3 × 10-8
inclination 21.982003 3 × 10-6 degrees
ascending node 14.813341 7 × 10-6 degrees
argument of perihelion 22.35365 1 × 10-5 degrees
mean anomaly 1.47253 1 × 10-5 degrees
9 Metis mean distance 2.386443361 2 × 10-9 AU
eccentricity 0.12115009 4 × 10-8
inclination 25.935090 3 × 10-6 degrees
ascending node 11.976524 9 × 10-6 degrees
argument of perihelion 63.84889 2 × 10-5 degrees
mean anomaly 352.35224 2 × 10-5 degrees
10 Hygiea mean distance 3.136204913 5 × 10-9 AU
eccentricity 0.11985212 4 × 10-8
inclination 24.617826 3 × 10-6 degrees
ascending node 351.002735 8 × 10-6 degrees
argument of perihelion 246.59314 2 × 10-5 degrees
mean anomaly 206.86683 2 × 10-5 degrees
15 Eunomia mean distance 2.644308703 2 × 10-9 AU
eccentricity 0.18701476 4 × 10-8
inclination 30.019997 4 × 10-6 degrees
ascending node 338.083505 7 × 10-6 degrees
argument of perihelion 50.17445 1 × 10-5 degrees
mean anomaly 296.45252 1 × 10-5 degrees
16 Psyche mean distance 2.921527397 6 × 10-9 AU
eccentricity 0.13756275 3 × 10-8
inclination 20.800688 3 × 10-6 degrees
ascending node 4.29608 1 × 10-5 degrees
argument of perihelion 15.45439 2 × 10-5 degrees
mean anomaly 188.69446 2 × 10-5 degrees
52 Europa mean distance 3.099221614 9 × 10-9 AU
eccentricity 0.10051921 5 × 10-8
inclination 19.562461 4 × 10-6 degrees
ascending node 17.54586 1 × 10-5 degrees
argument of perihelion 94.54815 3 × 10-5 degrees
mean anomaly 268.51717 3 × 10-5 degrees
65 Cybele mean distance 3.43189701 1 × 10-8 AU
eccentricity 0.10414563 7 × 10-8
inclination 20.251438 7 × 10-6 degrees
ascending node 4.19667 2 × 10-5 degrees
argument of perihelion 258.73968 4 × 10-5 degrees
mean anomaly 127.70237 4 × 10-5 degrees
511 Davida mean distance 3.17167262 1 × 10-8 AU
eccentricity 0.18235222 6 × 10-8
inclination 23.710076 5 × 10-6 degrees
ascending node 40.56063 1 × 10-5 degrees
argument of perihelion 49.29936 2 × 10-5 degrees
mean anomaly 48.08832 1 × 10-5 degrees
704 mean distance 3.064446939 8 × 10-9 AU
Interamnia eccentricity 0.14595014 4 × 10-8
inclination 31.363464 3 × 10-6 degrees
ascending node 325.795506 8 × 10-6 degrees
argument of perihelion 45.58638 2 × 10-5 degrees
mean anomaly 106.07912 2 × 10-5 degrees
The formal uncertainty in the elements in Table 7 is quite good. From the uncertainty in these osculating elements, the uncertainty in mean longitude at the epoch of integration and uncertainty in the mean motion of the asteroids are determined and given in Table 8.
| Asteroid | Uncertainty in Mean Longitude | Uncertainty in Mean Motion |
|---|---|---|
| (") | (" cen.-1) | |
| 1 Ceres | 0.05 | 0.023 |
| 2 Pallas | 0.05 | 0.024 |
| 3 Juno | 0.05 | 0.022 |
| 4 Vesta | 0.05 | 0.017 |
| 6 Hebe | 0.05 | 0.028 |
| 7 Iris | 0.04 | 0.024 |
| 8 Flora | 0.06 | 0.031 |
| 9 Metis | 0.11 | 0.040 |
| 10 Hygiea | 0.11 | 0.054 |
| 15 Eunomia | 0.06 | 0.034 |
| 16 Psyche | 0.11 | 0.076 |
| 52 Europa | 0.16 | 0.109 |
| 65 Cybele | 0.22 | 0.088 |
| 511 Davida | 0.09 | 0.137 |
| 704 Interamnia | 0.08 | 0.096 |
Comparing the uncertainty in mean longitude and mean motion with Table 2 of Standish (1986) shows that the uncertainties in these ephemerides compare very favorably with the uncertainties of the outer planets in DE200. Thus the formal errors give ephemerides that compare very favorably with DE200.
What then are the realistic uncertainties in the ephemerides? The least
squares adjustment of parameters assumes that the physical model has no
unaccounted for perturbations. The truth is all of these asteroids are in the
main belt and have much smaller masses than the planets. Hence, unaccounted
perturbations by other asteroids may cause departures from these ephemerides
that are potentially significantly worse than the uncertainties given in Table
8. Juno, as discussed below, is an example of unaccounted for perturbations.
Examination of Juno's ephemeris shows that the realistic uncertainties are, at
most, by a factor of five or so greater than the formal uncertainties.
RESIDUALS
Figures 5 through 19 shows the residuals in right ascension and declination
for the observations of each of the asteroid ephemerides. The red bar shows
the 3 sigma scatter in each twenty year era of observations. The number
in green is the number of observations included in that era.
Figure 5. Residuals for 1 Ceres.
Figure 6. Residuals for 2 Pallas.
Figure 7. Residuals for 3 Juno.
Figure 8. Residuals for 4 Vesta.
Figure 9. Residuals for 6 Hebe.
Figure 10. Residuals for 7 Iris.
Figure 11. Residuals for 8 Flora.
Figure 12. Residuals for 9 Metis.
Figure 13. Residuals for 10 Hygiea.
Figure 14. Residuals for 15 Eunomia.
Figure 15. Residuals for 16 Psyche.
Figure 16. Residuals for 52 Europa.
Figure 17. Residuals for 65 Cybele.
Figure 18. Residuals for 511 Davida.
Figure 19. Residuals for 704 Interamnia.
As expected, there is a drop in the root-mean-square value of the residuals over time. Those residuals from the early nineteenth century have a 3 sigma root-mean-square value of approximately 9" while the 3 sigma root-mean-square value for the last twenty years of the twentieth century is approximately 2". What is not expected is, aside from Ceres, Pallas, Juno, and Vesta, the root-mean-square value of the residuals varies widely between 1900 and 1960. There are two reasons for these root-mean-square values of the residuals.
First, the early twentieth century is a period during which few astrometric observations were made of most asteroids. As a result the statistics during this time period are poor. For example:
Second, During the early part of the twentieth century, several new
observatories, such as Bucharest, Athens, Purple Mountain, Santiago-San
Bernardo, and Madrid, began contributing observations that were significantly
worse, at least initially, than the older established observatories. As
described in Data Weighting, the observations
from these observatories were examined to make sure that they did not
contain systematic errors. In some cases, these observatories contributed a
large number of observations significantly increasing root-mean-square value of
the residuals for some eras. For example, the Bucharest Observatory
contributed 2863 observations; however, the root-mean-square value of the
residuals of the Bucharest observations was 5.1 times greater than similar
observations at other observatories during the same era.
Juno
Figure 7, the residuals for Juno, show residuals for Juno beginning in 1839
although Juno was discovered in 1804. No observations prior to 1839 were used
in determining the final ephemeris of Juno. The reason for cutting off the
observations prior to 1839 is shown in Figure 20, the residuals for Juno for
its entire observed history. There is an obvious systematic departure of the
residuals in right ascension between 1804 and 1839. Table 9 shows that the
mean residual in right ascension is large throughout the nineteenth century,
and is particularly large before 1840. The observations prior to 1830 are from
two different observatories. Observations from both observatories show the
same systematic drift in the residuals of Juno and they do not show systematic
drifts in the residuals for Ceres, Pallas, or Vesta. Hence, this deviation is
almost certainly caused by an encounter with an unmodeled asteroid during the
mid- to late-nineteenth century.
| Time Period | Mean Uncertainty in Right Ascension |
|---|---|
| (") | |
| 1804 - 1820 | 3.83 |
| 1821 - 1840 | 1.75 |
| 1841 - 1860 | 1.42 |
| 1861 - 1880 | 1.81 |
| 1881 - 1900 | 0.97 |
| 1901 - 1920 | 1.15 |
| 1921 - 1940 | -0.18 |
| 1941 - 1960 | -0.08 |
| 1961 - 1996 | 0.02 |
A search for perturbing asteroids showed close approaches by Psyche and Davida and an approach with a minimum distance of several tenths of an AU by Interamnia. Other large asteroids known to come within a few tenths of an AU of Juno during the nineteenth century are 24 Themis, 87 Sylvia, and 216 Kleopatra. However, inclusion of these bodies as perturbers was able to account for only 20% of the runoff in the ephemeris of Juno. Thus, to remove the effect of the unmodeled perturbation, the observations prior to 1839, the point at which the deviation of the mean residuals becomes greater than 1/3 sigma, were removed from the final ephemeris.
 |
| Figure 20. The complete residuals for 3 Juno. The red bars indicate the 3 sigma deviation for the residuals in each twenty year period, and the green numbers are the number of observations in that period. Before 1839 there is systematic slope to the residuals in right ascension before 1839. By 1807 the deviation from 0 is 2."1 or 2/3 sigma. |
The change in the initial conditions for Juno resulting from removing the data
prior to 1839 was approximately four times the formal uncertainty in the
initial conditions. This allows an estimate of the upper limits of the
realistic uncertainties with respect to the formal uncertainties in the
ephemerides. None of the other ephemerides show any obvious departures such as
Juno does. Thus it is likely that the effect of any unmodeled encounters for
the other asteroids produce changes in the orbits of the asteroids that result
in changes in the ephemerides of, at most, a few tenths of an arc second.
COMPARISON WITH OLD EPHEMERIDES
Hohenkerk (1997) has made a comparison of the ephemerides of Ceres, Pallas, Juno, and Vesta with the Duncombe (1969) ephemerides. The comparisons were made by comparing the apparent geocentric positions computed using the DE200 position of the Earth at daily intervals from Sept. 20, 1989 through Jan. 20, 2000. The results are presented in Figs. 21 - 24 and Table 10.
| Asteroid | Delta R.A. | Delta dec. | Delta dist. |
|---|---|---|---|
| (") | (") | (10-6 AU) | |
| 1 Ceres | -0.5 ± 0.2 | 0.03 ± 0.08 | 0.1 ± 1.8 |
| 2 Pallas | 0.1 ± 0.1 | -0.00 ± 0.06 | -0.2 ± 1.1 |
| 3 Juno | 0.2 ± 0.2 | -0.08 ± 0.36 | -0.3 ± 1.4 |
| 4 Vesta | 1.0 ± 0.2 | 0.04 ± 0.51 | -0.3 ± 4.6 |
Duncombe's ephemerides are old enough that at the time they were computed the only asteroid with a known mass was Vesta. Hence, there were no asteroid perturbations included in these ephemerides. The comparison with USNO/AE97 shows the effects of asteroid perturbations on these four large asteroids.
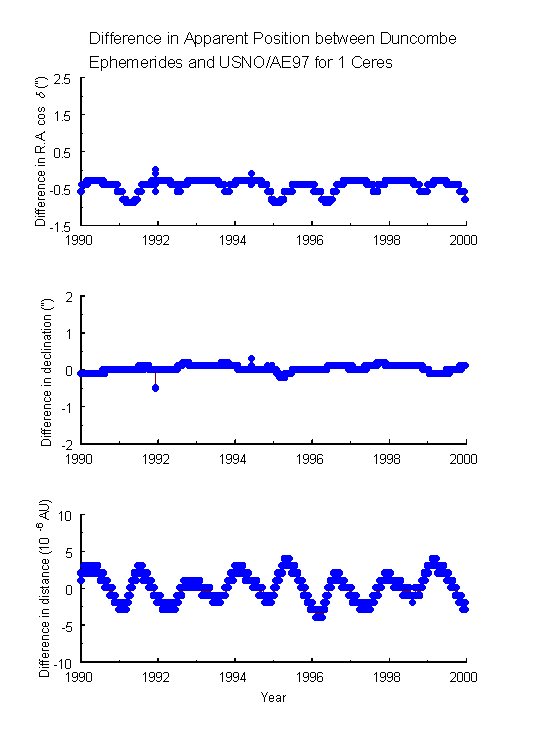 |
| Figure 21. The difference in apparent position between the Duncombe ephemeris and USNO/AE97 for 1 Ceres. |
Ceres, the most massive of the asteroids by a factor of 2.7, shows a systematic offset of -0."5 in right ascension and some minor fluctuations in right ascension, declination, and distance. These are the result of recent encounters with Vesta which has an orbital period near 3/4 that of Ceres. The last really close encounter between these two asteroids was in 1962, but they do approach to a few tenths of an AU about every 14 years.
 |
| Figure 22. The difference in apparent position between the Duncombe ephemeris and USNO/AE97 for 2 Pallas. |
Pallas has very little difference between the USNO/AE97 ephemeris and the Duncombe ephemeris. This is a result of its highly inclined, highly eccentric orbit which does not bring it within 1.5 AU of either Ceres or Vesta in this century.
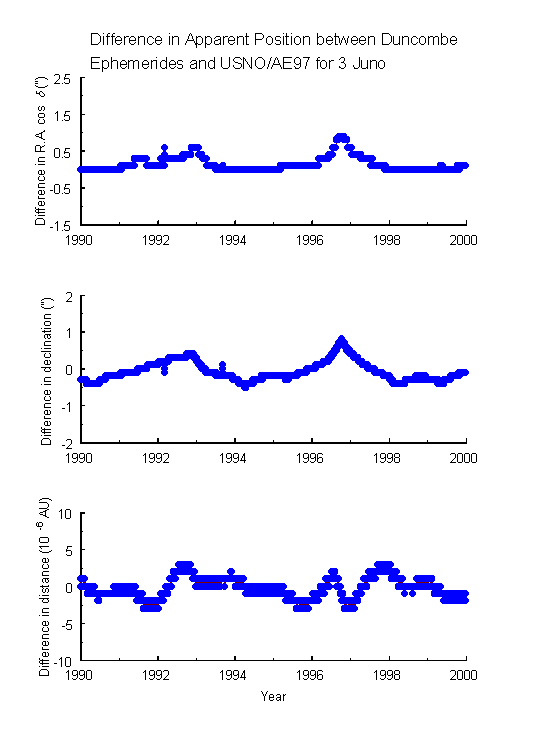 |
| Figure 23. The difference in apparent position between the Duncombe ephemeris and USNO/AE97 for 3 Juno. |
Juno shows two definite deviations from the Duncombe ephemerides. The first, small deviation is from an encounter with Pallas. The two asteroids did not get particularly close together, the minimum distance was several tenths of an AU, but they were reasonably close for nearly four years. The second, stronger deviation is the result of an encounter with 511 Davida. Notice that the peaks in the deviations of right ascension and declination do not coincide. The symmetry is broken because Juno approached Davida from below and behind, that is Juno started out on the south side of Davida's orbital plane as defined by the right hand rule and from a smaller mean longitude. Juno then passed in front of Davida after passing though Davida's orbital plane. The resulting encounter was symmetric enough that any long term effect from the encounter is too small to see at this scale. The symmetric nature of the perturbation of Juno by Davida is unfortunate because there is no reliable determination of the mass of Davida and the two year period of the deviation in Juno's orbit is too short to provide a good determination from existing data. An attempt to determine the mass of Davida from its perturbation of Juno resulted in a mass with an uncertainty of about 200%.
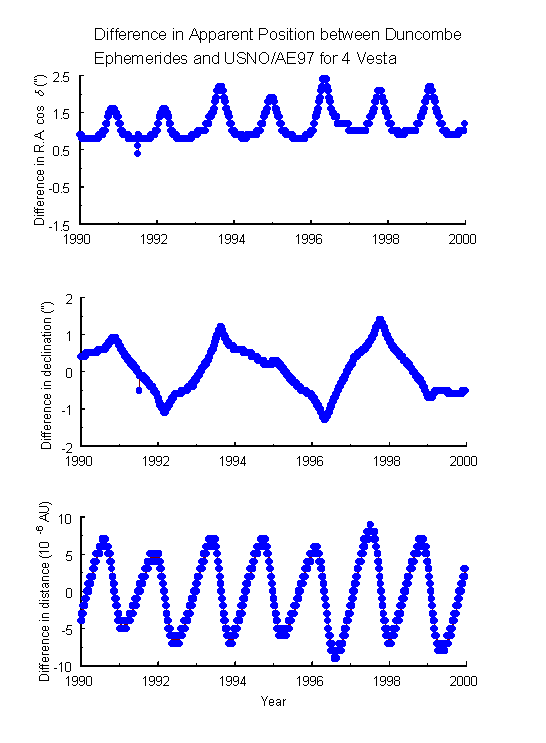 |
| Figure 24. The difference in apparent position between the Duncombe ephemeris and USNO/AE97 for 4 Vesta. |
Vesta shows the largest deviation from the Duncombe ephemerides. This is a
result of its frequent encounters with Ceres. The mean systematic deviation of
1."0 in right ascension is in the opposite direction as the systematic
deviation of Ceres and is nearly what would be naively expected from the ratio
of the mass of Vesta to the mass of Ceres. The sudden change in the
declination difference in 1999 is a result of the most recent encounter between
Vesta and Ceres.
Conclusions
A total of 59,258 optical and 2 radar observations were used to fit the ephemerides. Except for Juno the observations cover the period from the discovery of the asteroid to the most recent opposition with observations available. Observations for Juno prior to 1839 were not included because the residuals in preliminary ephemerides indicated that Juno has an unmodeled encounter with another large asteroid that resulted in a systematic drift between the ephemeris and the observations. Only those observations from 1839 or later were used to remove the effect of the unmodeled perturbation on the rest of the ephemeris.
As a part of improving the ephemerides, new masses were determined for Ceres, Pallas, and Vesta, the three largest asteroids. These masses are: Ceres = (4.39 ± 0.04) × 10-10 MSun, Pallas = (1.59 ± 0.05) × 10-10 MSun, Vesta = (1.69 ± 0.11) × 10-10 MSun. The mass for Ceres is smaller than most previous determinations of its mass. This smaller mass is a direct consequence of the increase in the mass determined for Pallas over previous determinations. The determination of the mass of Pallas from its effect on Ceres depends critically on the oldest, least accurate data. Hence it is desirable to make an independent determination of Pallas' mass. The mass determined by Standish & Hellings (1989) from Mars Viking observations is in accord with the mass determined here. Nor are there are any known good candidates that are significantly perturbed by Pallas.
The densities for these three asteroids are 2.05 ± 0.05 gm. cm-3 for Ceres, 4.2 ± 0.3 gm. cm-3 for Pallas and 4.3 ± 0.3 gm. cm-3 for Vesta. The density for Ceres is significantly greater than that of the taxonomically similar asteroid 253 Mathilde, or it may represent a difference between the C-type asteroids (Mathilde) and the G-Type asteroids (Ceres). This greater density likely represents a greater compaction of the far larger Ceres.
The internal accuracy of the ephemerides at epoch (Dec. 18, 1997 JD 2450800.5) ranges from 0."05 for Iris through 0."22 for Cybele and the uncertainty in the mean motion varies from 0."02 cen.-1 for Vesta to 0."14 cen.-1 for Davida. This compares very favorably with the internal errors for the outer planets in DE200. However, because the asteroids have relatively little mass and are subject to perturbations by other asteroids the actual uncertainties in their mean motions are no more than five times the internal error. Aside from Juno, there is no evidence of any large unmodeled perturbations, so their ephemerides should be good to an uncertainty of a few tenths of an arcsecond..
Comparison of the USNO/AE98 ephemerides with the older Duncombe ephemerides for the period of 1990 to 2000 of Ceres, Pallas, Juno, and Vesta show some differences. These differences can be mainly attributed to the perturbation of asteroids included in this model that were not included in the Duncombe ephemerides.
The author would like to acknowledge the contribution of Mr. Rahim
Taghizadegan for collecting the nineteenth century observations of the
asteroids from the Astronomische Nachrichten.
References
Adams A. N. & Scott D. K. 1964, Results of Observations with the Six-Inch Transit Circle 1956-1962, U. S. Naval Observatory Publications, 2nd Series, Vol. XIX, Part II
Adams, J. C. 1879, Astronomical Observations made at the Observatory of Cambridge, Vol. 21
Airy, G. B. 1831-1836, Astronomical Observations made at the Observatory of Cambridge, Vol. 3-8 Airy, G. B. 1837-1883, Astronomical Observations made at the Royal Observatory, Greenwich, 1836-1881
Alvarez del Castillo, E. M., Sykes, M. V., Davis, D.R. & Tholen, D. 1993, An Interactive Database for Asteroids, Bull. Amer. Astron. Soc., 25, 1127.
Ash, M. E. 1965, Generation of Planetary Ephemerides on an Electronic Computer, Lincoln Laboratory, Tech. Report 391
Astronomische Nachrichten 1823-1900, 1-150
Baillaud, B. 1910-1911, Annales de l'Observatoire de Paris, 1892-1893
Birlan, M., Baruccii, M. A., & Fulchignoni, M. 1996, G-Mode Analysis of the Reflection Spectra of 84 Asteroids, Astron. Astrophys., 305, 984-988
Blackwell, K. C., Buontempo, M. E., Eldridge, P., & Swifte, R. H. D. 1975, Provisional Positions of the Sun, Moon, and Planets, Royal Observatory Bulletins, No. 180
Bowell, E. 1994a, MPC 24219
Bowell, E. 1994b, MPC 24084
Bowell, E. 1994c, MPC 24085
Bowell, E., Turnbull, M., Koehn, B., & Muinonen, K. 1996, Orbital and Ephemeris Accuracy of Multi-Apparition Asteroids, poster paper presented at Asteroids, Comets, & Meteors '96
Buontempo, M. E., Carey, J. V., & Eldridge, P. 1973, Provisional Positions of the Sun and Planets 1957-1971, Royal Observatory Bulletins, No. 178
Carlsberg Meridian Catalog, Numbers 1 - 9 1984 - 1995, Copenhagen University Observatory, Royal Greenwich Observatory, Real Instituto y Observatorio de la Armada en San Fernando
Challis, J. 1837-1864, Astronomical Observations made at the Observatory of Cambridge, Vol. 9-20
Christie, W. H. M. 1884-1902, Astronomical and Magnetical and Meteorological Observations made at the Royal Observatory, Greenwich, 1882-1900
Christie, W. H. M. 1903-1912, Astronomical and Magnetical and Meteorological Observations made at the Royal Observatory, Greenwich, 1901-1910
Drummond, J. D. & Cocke, W. J. 1988, Triaxial Ellipsoid Dimensions and Rotational Pole of 2 Pallas from Two Stellar Occultations, Icarus, 78, 323 - 329
Duncombe, R. L. 1969, Heliocentric Coordinates of Ceres, Pallas, Juno, Vesta 1928 - 2000, Astronomical Papers Prepared for the Use of the American Ephemeris and Nautical Almanac, Vol. XX, Pt II
Dyson, F. W. 1913-1933, Astronomical and Magnetical and Meteorological Observations made at the Royal Observatory, Greenwich, 1911-1932
Dyson, F. W. & Jones, H. S. 1934, Observations made at the Royal Observatory, Greenwich in the Year 1933
Folkner, W. M., Charlot, P., Finger, M. H., Williams, J. G., Sovers, O. J., Newhall, X X, & Standish, E. M., Jr. 1994, Determination of the Extragalactic-Planetary Frame Tie from Joint Analysis of Radio Interferometric and Lunar Laser Ranging Measurements, Astron. Astrophys., 287, 279-289
Gillis, J. M. 1846, Astronomical Observations made at the Naval Observatory, Washington
Gillis, J. M. 1867, Astronomical Observations made During the Years 1851 and 1852, at the U.S. Naval Observatory
Goffin, E. MPC 22573
Goffin, E. MPC 22386
Harkness, W. & Skinner, A. N. 1900, Transit Circle Observations of the Sun, Moon, Planets, and Miscellaneous Stars 1894-1899, Publications of the U.S. Naval Observatory 2nd Series, Vol. I
Henderson, T. 1839-1847, Astronomical Observations made at the Royal Observatory, Edinburgh, Vol. 2-6
Henderson, T. & Smythe C. P. 1848-1852, Astronomical Observations made at the Royal Observatory, Edinburgh, Vol. 7-10
Herget, P. 1947, Minor Planet Circular, 1
Hilton, J. L. 1997, The Mass of the Asteroid 15 Eunomia from Observations of 1313 Berna and 1284 Latvia, Astron. J., 114, 402 - 408
Hilton, J. L., Seidelmann, P. K., & Middour, J.
1996, Prospects for Determining Asteroid Masses, Astron. J.,
112, 2319 - 2329
Hipparcos Catalogue No. 1 1997, , Vol. 1, section
2.7.2, 242-243
Hohenkerk, C. 1997, Her Majesty's Nautical Almanac
Office, private communication
Hudson, R. S. & Ostro, S. J. 1995, Science,
270, 84
Hughes J. A. & Scott D. K. 1982,
Results of Observations with the Six-Inch Transit Circle 1963-1971,
U. S. Naval Observatory Publications, 2nd Series, Vol. XXIII, Part III
Hughes J. A., Scott D. K., & Branham, R. L. 1992,
Results of Observations with the Seven-Inch Transit circle
1967-1973, U. S. Naval Observatory Publications, 2nd Series, Vol.
XXVI, Part II
Jones, H. S. 1935-1953, Observations made at
the Royal Observatory, Greenwich, 1934-1940
Koller, M. 1833-1839 Astronomische
Nachrichten, 10-16
Kuzmanoski, M. 1995, A Method for Asteroid
Mass Determination, IAU Symposium No. 172: Dynamics, Ephemerides, and
Astrometry in the Solar System, Paris, France
Lambert, J. V. 1985, Occultation and Lightcurve
Analysis: The Figure of 2 Pallas, Ph D Thesis, New Mexico State U.
Landgraff, W. 1992, A Determination of the
Mass of (704) Interamnia from Observations of (903) Moultona, IAU
Symposium No. 152: Chaos, Resonance, and Collective Dynamical Phenomena in the
Solar System, S. Ferraz-Mello ed., (Kluwer: Dordrecht, the Netherlands),
179 - 182
Le Verrier, U. -J. 1858-1867, Annales de
l'Observatoire Imperial de Paris, Vol. 1-22
Loewy, M. 1898-1907, Annales de
l'Observatoire de Paris, 1889-1891
MacLear, T. & Stone, J. E. 1871-1872,
Results of Astronomical Observations made at the Royal Observatory, Cape of
Good Hope, 1856-1858
MacLear, T. & Gill, D. 1900, Results of
Astronomical Observations made at the Royal Observatory, Cape of Good Hope,
1866-1879
Magnuson, P. 1986, Distribution of Spin Axes and
Sense of Rotation for 20 Large Asteroids, Icarus, 68, 1
Maskelyne, N. 1811, Astronomical Observations
made at the Royal Observatory at Greenwich from 1799 to 1810
Marsden, B. 1991a, Minor Planet Circular, 18,847
Marsden, B. 1991b, Minor Planet Circular, 17,474
Maury, M. F. 1846-1859, Astronomical
Observations made at the U.S. National Observatory, Washington, Vol. 1-5
Merline, W. J., Stern, S. A., Binzel, R. P.,
Festou, M. C., Flynn, B. C., & Lebofsky, L. A., 1996, HST Imaging of 1
Ceres, Bull. Am. Astron. Soc., 28, 1025
Millis, R. L., Wasserman, L. H., Franz, O. G., Nye,
R. A., et al. 1987, The Size Shape, Density, and Albedo of Ceres from Its
Occultation of BD+8° 471, Icarus, 72, 507 - 516
Minor Planet Center 1997, Minor Planet Center Observation
Retrieval System
Morrison, L. V., Argyle, R. W., Helmer, L.,
Fabricius, C., Einicke, O. H., Quijano, L., & Muinos, J. L. 1990,
Optical Reference Frame Defined by Carlsberg Meridian Catalogue La Palma
Number 4, IAU Symposium No. 141: Inertial Coordinate System on the Sky,
J. H. Lieske & V. K. Abalakin eds.,
Mouchez, C. -A. 1880-1892, Annales de
l'Observatoire de Paris, Vol. 24 & 1870-1884
Newhall, X X 1989, Numerical Representation of
Planetary Ephemerides, Celestial Mechanics , 45, 305 - 310
Ostro, S. J., Campbell, D. B., Chandler, J. F.,
Shapiro, I. I., Hine, A. A., Velez, R., Jurgens, R. F., Rosema, K. D., Winkler,
R., & Yeomans 1991, Asteroid Radar Astrometry, Astron. J.,
102, 1490 - 1502
l'Observatoire Imperial de Paris, 1871, Annales
de l'Observatoire Imperial de Paris, Vol. 23
Pond, J. 1815-1835, Astronomical Observations
made at the Royal Observatory at Greenwich, 1811-1835
Reslhuber, A. 1841-1870, Astronomische
Nachrichten, 18-75
Schubart, J 1976, New Reduction and Collection
of Meridian Observations of Ceres and Pallas, Astron. Astrophys.
Suppl., 26, 405
Standish, E. M., Jr. 1987, Ephemeris Comparison
- PEP740 vs. DE118, Jet Propulsion Laboratory Interoffice Memorandum IOM
314.6-799
Standish, E. M., Jr. 1986, Numerical Planetary
and Lunar Ephemerides: Present Status, Precision, and Accuracies, IAU
Symposium No. 114: Relativity in Celestial Mechanics and Astrometry, J.
Kovalevsky and V. A. Brumberg eds. (Dordrecht, Holland: Reidel), 71 - 83
Standish, E. M., Jr. 1990, The Observational
Basis for JPL's DE200, the Planetary Ephemerides of the Astronomical
Almanac, Astron. Astrophys., 233, 252 - 271
Standish, E. M., Jr. & Hellings, R. W. 1990,
, A Determination of the Masses of Ceres, Pallas, and Vesta from Their
Perturbations on Mars, Icarus, 80, 326 - 333
Standish, E. M. Jr., Newhall, X X, Williams,
J. G., & Folkner, W. M. 1995, JPL Planetary and Lunar Ephemerides,
DE403/LE403, Jet Propulsion Laboratory Interoffice Memorandum IOM
314.10-127
Stone, R. 1995 - 1997a, U.S. Naval Observatory,
Flagstaff Station, FASST observations, private communication
Stone, R. 1997b, CCD Astrometry of Asteroids in
the Extragalactic Reference Frame, Astron. J., 113, 2317 -
2324
Stoy, R. H. 1968 Second Cape Catalog for 1950.0:
Observations of the Sun, Moon, and Planets, Annals of the Cape
Observatory, Vol. XXIII
Strasser, G. 1870-1880, Astronomische
Nachrichten, 76-97
Tedesco, E. F. 1989, Asteroid Magnitudes, UBV
Colors, and IRAS Albedos and Diameters, Asteroids II, R. P. Binzel,
T. Gehrels, and M. S. Matthews eds. (Tucson, U. of Arizona Press) 1090 - 1138
Tedesco, E. F. 1992, IRAS Minor Planet
Survey, Astronomical Data Center, holding 2190
Tholen, D.J. 1989, Asteroid Taxonomic
Classifications, in Asteroids II, R. P. Binzel, T. Gehrels, and M.
S. Matthews eds., (Tucson, U. of Arizona Press) 1139-1150
Thomas, P. C., Binzel, R. P., Gaffey, M. J.,
Storrs, A. D., Wells, E. N., & Zellner, B. H. 1997, Impact Excavation on
Asteroid 4 Vesta: Hubble Space Telescope Results, Science,
277, 1492
Tisserand, F. 1893-1896, Annales de
l'Observatoire de Paris, 1885-1888
Tucker, R. H., Buontempo, M. E., Gibbs, P., &
Swifte, R. H. D. 1983, Third Greenwich Catalogue of Stars, Sun, Planets,
and Moon for 1950.0, Royal Greenwich Observatory Bulletins, No. 187
U.S. Naval Observatory 1906a, Transit Circle
Observations of the Sun, Moon, Planets, and Miscellaneous Stars 1900-1903,
Publications of the U.S. Naval Observatory 2nd Series, Vol. IV, Part I
U.S. Naval Observatory 1906b, Transit Circle
Observations of the Sun, Moon, Planets, and Comets 1866-1891,
Publications of the U.S. Naval Observatory 2nd Series, Vol. IV, Part II
Viateau, B. & Rapaport, M. 1997, The
Bordeaux Meridian Observations of Asteroids. First Determination of the Mass of
(11) Parthenope, Astron. Astrophys., 320, 652 - 658
Veverka, J., Bell, J. F., III, Chapman, C., Malin,
M., McFadden, L. A., Murchie, S., Robinson, M., Thomas, P. C., Yeomans, D. K.,
Harch, A. Williams, B. G., Clark, B., Farquhar, R. W., Cheng, A., & Dunham, D.
W. 1997, NEAR's Flyby of Mainbelt Asteroid 253 Mathilde, Bull.
Am. Astron. Soc., 29, 958
Watts, C. B. & Adams, A. N. 1949,
Results of Observations with the Six-Inch Transit Circle 1925-1941,
U. S. Naval Observatory Publications, 2nd Series, Vol. XVI, Part I
Williams, J. G. 1984, Determining Asteroid
Masses from Perturbations of Mars, Icarus, 57, 1 - 13
Williams, J. G. 1984, Asteroid Family
Identifications and Proper Elements, Asteroids II, R. P. Binzel,
T. Gehrels, and M. S. Matthews eds. (Tucson, U. of Arizona Press) 1034 - 1072
Yarnall, M., Major, J., Robinson, T. J. 1872,
Results of Observations made at the United States Naval Observatory with the
Transit Instrument and Mural Circle in the Years 1853 to 1860, Inclusive,
Washington Observations for 1871 - Appendix II
Yeomans, D. K., Chodas, P. W., Keesey, M. S.,
Ostro, S. J., Chandler, J. F., & Shapiro, I. I. 1992, Asteroid and Comet
Orbits Using Radar Data, Astron. J., 103, 303 - 317